Use this PowerPoint listing the properties of quadrilaterals – including angles, equal/parallel sides, line and rotational symmetry and diagonals – as part of your teaching on geometry and measures.
Some slides/ideas taken from other resources include:
- Thanks to @Owen134866 for the starter slide, which the rest of the PowerPoint is based on (Source)
- Thanks to @Bademola for the riddle questions
Quadrilaterals in the curriculum
Understanding the properties of quadrilaterals is a fundamental aspect of the KS3 maths curriculum. Quadrilaterals are four-sided polygons with various properties and classifications, which pupils are expected to learn.
Types of quadrilaterals
Firstly, KS3 students should recognise and differentiate between various types of quadrilaterals, including:
- Squares: Four equal sides, four right angles, opposite sides are parallel.
- Rectangles: Opposite sides are equal and parallel, four right angles.
- Parallelograms: Opposite sides are equal and parallel, opposite angles are equal.
- Rhombuses: Four equal sides, opposite angles are equal, opposite sides are parallel.
- Trapeziums: At least one pair of parallel sides.
- Kites: Two pairs of adjacent sides are equal, one pair of opposite angles are equal.
Properties and relationships
- Angles: The sum of the interior angles in any quadrilateral is always 360 degrees. This can be derived from the fact that a quadrilateral can be divided into two triangles, each with angles summing to 180 degrees.
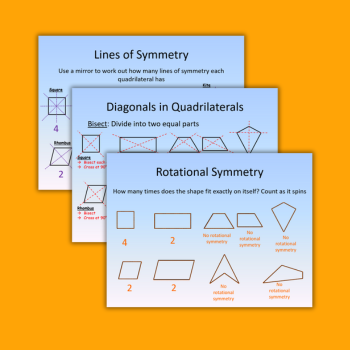
- Symmetry: Different quadrilaterals exhibit varying degrees of symmetry. For instance, squares and rectangles have both line and rotational symmetry, while parallelograms typically only have rotational symmetry.
- Diagonals: The diagonals of quadrilaterals have specific properties. In squares and rectangles, they are equal and bisect each other at right angles. In rhombuses, they also bisect at right angles but are not equal. In kites, one diagonal is the axis of symmetry.
Problem solving
Students should also be able to apply their knowledge of these properties to solve problems. For example calculating missing angles or sides, understanding symmetry, and using properties to classify quadrilaterals. Practical tasks might include drawing quadrilaterals with given properties or using coordinate geometry to verify properties.
Real-world applications
Understanding quadrilaterals extends to real-world contexts, such as architecture and engineering, where recognising these shapes and their properties is crucial. This foundational knowledge is also essential for advanced mathematical concepts and practical applications.
Fiona Ryan is a secondary maths teacher. You can find her resources on her TES page fionaryan88.