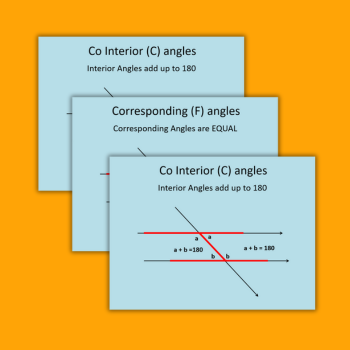
This PowerPoint on angles of parallel lines shows alternate, corresponding and interior angles, followed by some questions to practise.
It’s ideal for KS3 and GCSE students.
Angles of parallel lines in the curriculum
In the KS3 maths curriculum, understanding the angles of parallel lines is a crucial component. It lays the foundation for higher-level geometry and various practical applications. This topic is integral for developing pupils’ spatial reasoning, problem-solving abilities, and logical thinking. These skills are essential both in mathematics and across other subjects.
Conceptual understanding and reasoning
Teaching the angles of parallel lines helps pupils grasp the properties of geometric shapes and the relationships between angles. Key concepts such as corresponding angles, alternate interior angles, and co-interior angles (also known as allied or consecutive angles) become the building blocks for understanding more complex geometrical theorems and proofs. This understanding is fundamental for progressing to further topics such as:
- the properties of polygons
- trigonometry
- circle theorems.
Practical applications for angles of parallel lines
The principles of parallel lines and their angles have numerous practical applications. For instance, in design and engineering, understanding these concepts is essential for creating accurate plans and models. In everyday contexts, this knowledge helps in tasks like reading maps, and understanding the design of roads and railways. It’s even useful in art and architecture, where perspective and symmetry are crucial.
Problem-solving and logical thinking
Exploring the angles of parallel lines encourages logical thinking and problem-solving skills. Pupils learn to apply geometric rules and properties to find missing angles, which enhances their ability to approach and solve problems systematically. This analytical approach is beneficial not only in mathematics but also in science, technology, and even in developing critical thinking skills for real-life situations.
Curriculum integration and progression
In the KS3 curriculum, the study of parallel lines and angles is strategically placed to ensure a smooth transition from basic geometric concepts covered in primary school to more advanced topics in secondary education. By mastering these foundational concepts, pupils are better prepared for the complexities of GCSE mathematics and beyond. This topic also integrates well with algebra. Pupils often use algebraic expressions to represent angle relationships, reinforcing their algebraic manipulation skills.
This foundational knowledge equips pupils with critical skills to pave the way to success in more advanced mathematical studies and various practical fields.
Ben Cooper is primary principal at GEMS Wellington Al Khail, a UK curriculum school in Dubai. See more of his work on X (formerly Twitter) @WAGOLLTeaching