KS3 Geometry – What is an angle?
Angles crop up in a lot of topics in mathematics, but students are often confused about what exactly an angle is…
- by Colin Foster
In this lesson, students go back to basics on angles and think about what makes one angle bigger or smaller than another.
Which angle is largest?
This task is intended to bring to the surface possible confusion over angles: What does ‘angle’ mean? This is a hard thing to explain in words, and students’ answers may include words like ‘distance’ or ‘how far’, which may indicate confusions that this lesson will address.
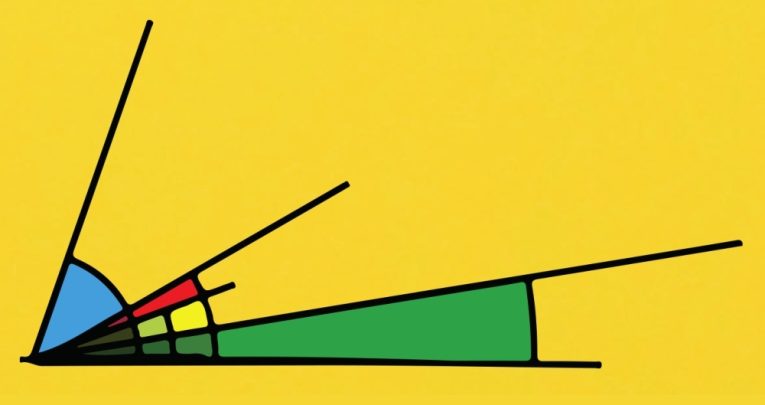
There is no need to try to resolve these yet or provide a better definition – that will come later. Look at the drawings below. Which one shows the biggest angle?
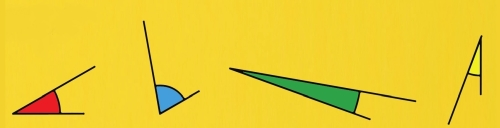
Students may find this difficult, because it’s quite hard to compare the angles when they are presented in different orientations. But they may also find it hard to compare the angles because they confuse angle with other features, such as the lengths of the arms, the arc length or the shaded areas.
Integer multiples
The best way to resolve this kind of difficulty is to take the time to print these four angles onto paper [a resource sheet containing these is available here] and allow students to cut them out.
Once the angles are separated, students can reorient them so that they all have one arm pointing in the same direction, as below.
Now, students should be able to decide the order, at least of Red, Blue and Green, but they may still be unsure or wrong about Green and Yellow.
If so, the other really useful thing about cut-outs, if they are trimmed right down to the arms, is that they can then be placed on top of each other, and this should resolve any remaining uncertainties.
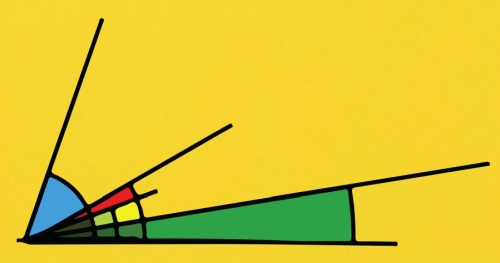
Now, students should be able to see that Green < Yellow < Red < Blue, and they may be quite surprised with aspects of this order – particularly that Green < Yellow.
In addition, students may realise that all four angles are all integer multiples of the green angle, which is actually 10°. By moving this around inside the other angles, students should be able to determine that Yellow = 2Green, Red = 3Green and Blue = 7Green; or, Yellow = 20°, Red = 30° and Blue = 70°.
What it is and isn’t
Conclude the lesson by asking students the same question as at the start: What does ‘angle’ mean? They may still find it difficult to give a good definition (eg that it is a measure of how much something has turned), but they should at least be clearer about what an angle isn’t.
You could ask: Why is ‘the distance between two lines’ not a good explanation of angle? And encourage them to critique that attempt, perhaps using Red, Blue, Green and Yellow to help.
Colin Foster (@colinfoster77) is a Reader in Mathematics Education at the Mathematics Education Centre at Loughborough University. He has written many books and articles for mathematics teachers – find out more at foster77.co.uk
