Pythagoras’ theorem questions – What students often get wrong about triangles
When using Pythagoras’ Theorem, students will often muddle up calculating a hypotenuse with calculating a leg, notes Colin Foster…

- by Colin Foster
- Reader in mathematics education at Loughborough University Visit website
In this Pythagoras’ theorem questions lesson, students distinguish a hypotenuse from a leg by repeatedly switching between them.
Pythagoras’ theorem questions – the difficulty
What’s the same and what’s different about these two triangles?
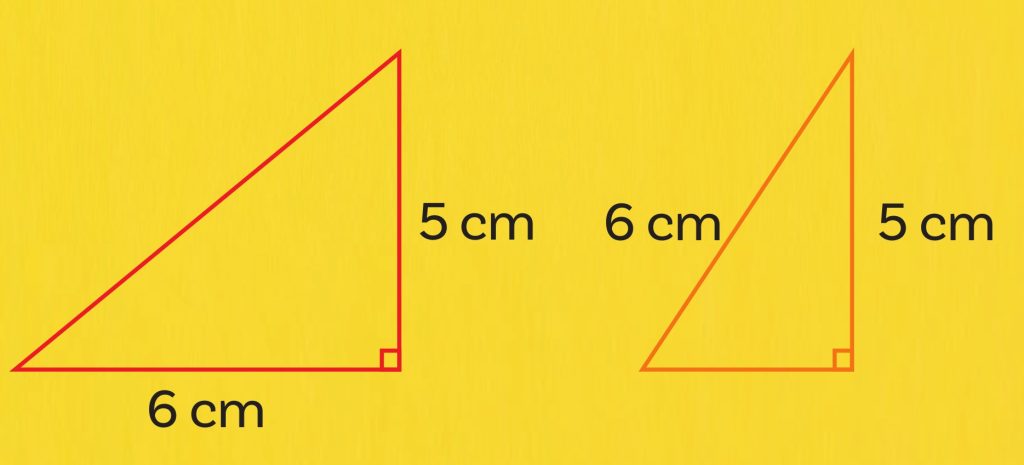
Students might pick up on the different colours, that they are both right-angled, that the two given lengths are the same but differently placed (6 cm is a leg in the red triangle and a hypotenuse in the orange triangle), and that the areas and perimeters are different.
Which triangle has the larger perimeter?
It isn’t necessary to do any calculations to reason that the red triangle is larger than the orange one. The largest side in a right-angled triangle is always the hypotenuse. In the orange triangle, this is 6 cm, so the perimeter is 6 + 5 + something smaller than 6 cm. In the red triangle, the perimeter is 6 + 5 + something larger than 6 cm. So, the red triangle has a larger perimeter.
Work out the perimeters of these triangles.
The missing sides are
red: [square root of] (6² + 5²) = [square root of] 61 = 7.810 cm (3 dp)
orange: [square root of] (6² – 5²) = [square root of] 11 = 3.317 cm (3 dp)
So the perimeters are
red: 18.810 cm (3 dp)
orange: 14.317 cm (3 dp)
Watch out for students being unsure about whether they need to square and add or square and subtract.
The solution
With the red and orange triangles, we could see without calculating which one must have the larger perimeter. But that isn’t always the case. Which of these triangles has the larger perimeter?
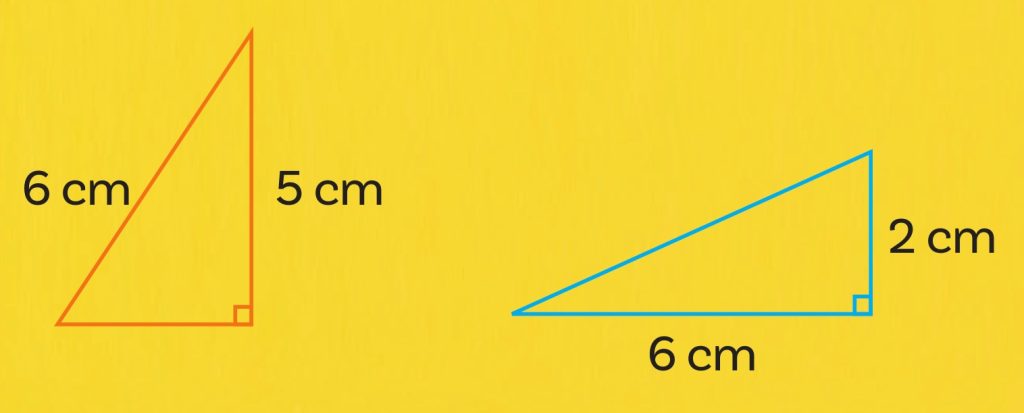
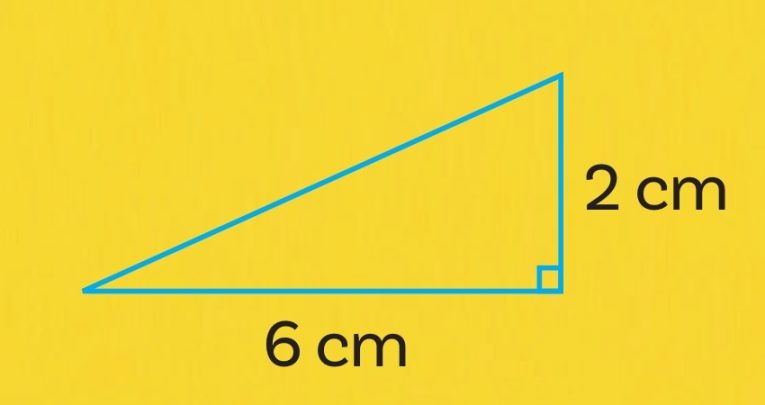
Students might compare the 5 cm with the 2 cm and conclude that the orange one has the greater perimeter, but this is wrong because the 6 cm is the hypotenuse in the orange triangle but a leg in the blue triangle.
This time we need to calculate. We already know the perimeter of the orange triangle, but the blue one is new, and its perimeter comes to [square root of] (6² + 2²) + 6 + 2 = 14.325, which is just slightly longer than the orange perimeter – too small to detect by eye.
Now try these two:
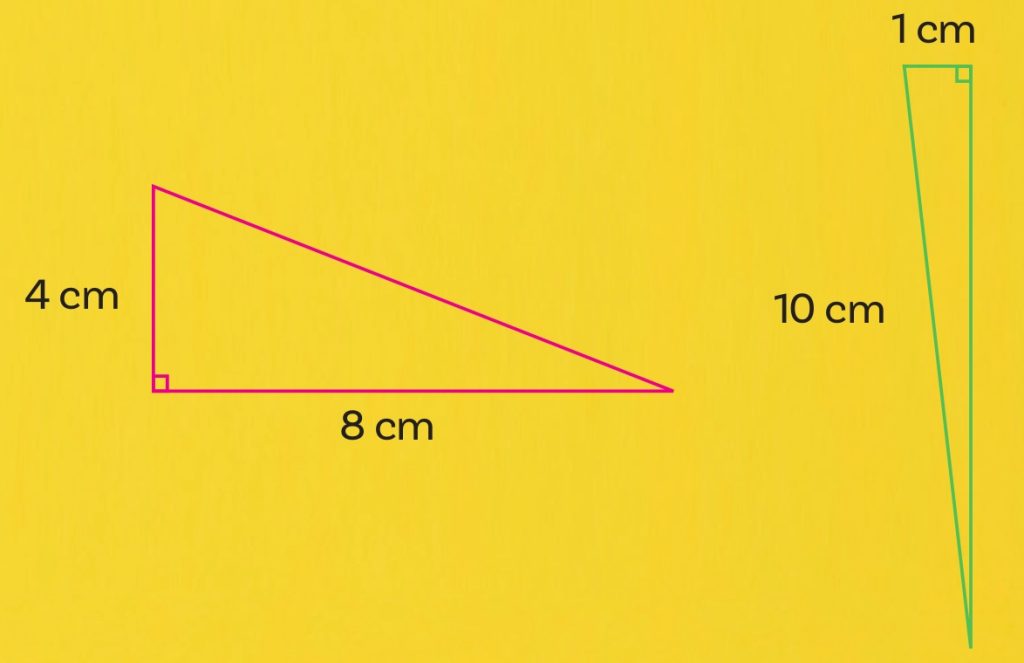
These are very close: pink perimeter = 20.944 cm and green perimeter = 20.950 cm (3dp). Students might struggle to see that the 10 cm side in the green one is a hypotenuse and not a leg. It must be the hypotenuse because it is opposite the right angle.
Checking for understanding
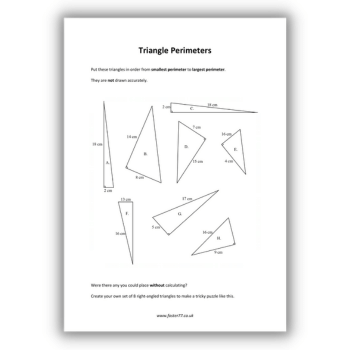
To assess students’ understanding of Pythagoras calculations, use this Pythagoras’ theorem questions and answers worksheet. Ask them to order the triangles by perimeter, starting with the smallest.
The answer is C < A < B < H < G < F < E < D.
Colin Foster (@colinfoster77) is a Reader in Mathematics Education in the Department of Mathematics Education at Loughborough University. He has written many books and articles for mathematics teachers; find out more foster77.co.uk










