GCSE maths – Strategies to help your pupils succeed

It’s debatable whether maths is the cause of more anxiety and exam jitters than other GCSE subjects – but what’s certain is that students will benefit hugely from following a focused and robust exam preparation plan…

Why GCSE maths matters

In the general pecking order of GCSE subjects, maths enjoys something of a privileged position.

A core subject alongside English and science, maths is a centuries-old discipline that’s evolved to a point where an understanding of basic mathematical concepts is vital in order to successfully live, work and prosper in the modern world.

More specifically, many post-16 destinations require GCSE maths grade 4 pass and above for further study and careers development.

It’s one of the most important qualifications your students study for, and they know it. This is why GCSE maths exams tends to loom large in students’ minds.

As any maths teacher will know, of course, there is beauty and wonder aplenty to be found in the study of maths – not to mention any number of practical life skills that might not readily apparent to your students. It’s your job to make these explicit in a range of creative and imaginative ways.

Students will enter Y10 with a certain set of ingrained attitudes and habits towards maths, but that doesn’t mean they’re set in stone.

As the prospect of taking their maths GCSE becomes increasingly real, your students’ may well come to treat the subject with a newfound seriousness that could serve them well when it comes to their revision.

Let’s begin with a look at how to build on what your students have learnt so far and how to bring about a change in attitude where needed…

---

Make GCSE maths relevant

The GCSE maths paper your students will, of course, largely consist of abstract questions intended to test their knowledge and understanding of key mathematical concepts, interspersed with a handful of ‘real world’ problems.

By the time they’ve reached KS4, students will know why it’s important to pass their GCSE maths exam, and therefore see the lesson content they’re learning as a means to that end.

Most will fail to see why these particular concepts are so important, and struggle to relate algebraic calculations to their everyday lives.

Ideally, your students will have already received a fuller appreciation before now of maths’ daily importance to all our lives, but it’s not too late to lay the groundwork now if needs be.

1. Focus on real-world applications

Some maths topics lend themselves especially well to meaningful, real-world contexts, such as arithmetic, ratio and proportion and geometry.

One obvious example is DIY – are there any photos or videos you can show your class to illustrate how you calculated various measurements and positionings?

2. Use maths problems with care

Consider the following: “Tim has x sweets. John has 3 more than Tim. Together they have 15 sweets. How many does John have?”

The question is essentially asking students to respond with a neat equation, but by trying to present this as a real-world problem that exists within the students’ frame of reference, they may well try and use their own real-world strategies to try and answer it.

Questions like this can be useful – the ability to construct and solve equations for given problems is a crucial skill. However, we need to make it clear that that’s what the question is intended to do.

3. Harness those cross-curricular links

If your students want a ‘real life’ example of when they’ll need to use certain maths skills, the physics lesson they have scheduled later in the day would certainly count. The same may well apply to students’ experiences in geography, D&T and PE lessons.

Make these links more explicit by devising a school-wide calculation policy. This involves different subject teachers modelling the processes used in your school’s maths classrooms.

That way, students will soon start to recognise just how relevant and versatile the GCSE-level maths they’ve been learning really is.

4. Remind students that their skills are transferable

That said, you could argue that some elements of the standard GCSE curriculum won’t be especially relevant to your students’ future working lives. Not all of them will require knowledge of trigonometric ratios, or the ability to solve quadratic equations.

Even in the process of learning these seemingly arcane subjects, however, your students will still be picking up a range of useful transferable skills. This includes the ability to think logically and follow a specific thought process or method, for example. Critical thinking is another useful skill, along with oral and written communication skills.

The tasks students perform when studying algebra – such as solving equations, expanding and factorising – are especially useful in the context of computer programming.

Make it clear to your students that it’s because they possess these kinds of transferable skills that a number of different industries seek out mathematics graduates.

5. Share the ‘awe and wonder’ of mathematics

Your GCSE students will be studying maths in what’s undeniably a target-driven environment – there are specific curriculum areas you’ll need to cover (see below) and certain grades they’ll need to reach at a set time.

This can lead to us presenting GCSE maths as a series of fragmented, almost arbitrary skills that they ‘need to know’, without making it clear why.

Even with all this going on, teachers can still model maths as something enjoyable in and of itself. The ideal time to do this is arguably at KS3. Here you can introduce students to areas outside the curriculum, such as Fermat’s Last Theorem and set theory in novel and engaging ways.

At KS4, it’s still worth encouraging students to see maths as a valuable tool for engaging with and questioning the world around them. This can be everything from why a thrown ball moves in the way it does, to weather patterns and how digital devices render graphics.

With your students sufficiently motivated to study and revise, the next step is to ensure that they’re able to comprehend and retain the knowledge they’ll need to succeed come exam time. And for that, it can be useful to…

---

Help your students think like mathematicians

Whichever form of GCSE maths assessment your students undergo, it will at some point involve them answering some tricky questions that could cause them to come unstuck. 

As maths teacher Craig Barton, founder of Mr Barton Maths, notes, the process of preparing students to confidently tackle a GCSE past paper or similar form of assessment should involve developing their readiness to conjecture, hypothesis and problem solve.

You can do this through careful sequencing when it comes to GCSE maths revision. Instead of presenting students with a series of random, disconnected practice questions and working through them, have each question build upon the previous one by keeping key aspects constant and changing just one or two elements – the basis of an approach that Barton calls Reflect, Expect, Check, Explain.

The Reflect, Expect, Check, Explain model

When a student’s GCSE maths revision arrives at fractions, for example, Q1 of their practice questions might ask students to add two fractions together and work out the answer.

Q2 might then change one element – perhaps the numerator or denominator of either fraction. The purpose of this is to show students that the questions aren’t unrelated, but that there’s something else at play that they need to pick up on.

We can break down the ‘reflect, expect, check explain’ process as follows: 

• What’s the same and what’s different about this question? (Reflect) 
• What do I think will happen to the answer? (Expect) 
• Am I right? (Check) 
• If not, can I figure out why? 
• If yes, can I explain why to someone else? (Explain)

Not every student will spot the intended relationships between the questions straight away. However, all will benefit from thinking that little bit deeper about the maths revision task you’ve set them.

Intelligent practice

You can get a better sense of the intention behind the ‘Reflect, Expect, Check, Explain’ model by contrasting it against more traditional approaches to compiling GCSE maths revision questions.

This will typically involve setting fairly easy questions to start with. These then get progressively harder. Simultaneously, you’re calling on students to demonstrate more sophisticated – and crucially, more interesting – mathematical techniques.

At first glance this makes sense, with mastery of the basics normally required before moving on to more complex activities.

What this means, however, is that every student will encounter the more boring, routine mathematics early on, while only some will reach the part where things get interesting.

Barton’s ‘ Reflect, Expect, Check, Explain’ sequences can and do get gradually harder as they go along. However, at every stage they’re prompted to compare the elements of adjacent questions, spot what’s different, interrogate the reasons why that might be and explain their reasoning.  

When students work through a series of disconnected maths revision questions, they have little reason to think any further about questions they’ve answered correctly.

When the questions are sequenced thoughtfully, every student will have an incentive to consider why they’ve been able to answer a question correctly. They’ll reflect on the substance of what they’re revising more deeply.

---

GCSE maths revision classes – what not to do

We’ve covered how to keep students engaged and structure maths revision activities in a way that encourages deeper engagement with the topic at hand.

But what about those traditional class activities that could potentially do more harm than good? In Craig Barton’s view, these are the maths revision habits and practices that teachers would be best off avoiding:

1. Using past papers in every lesson

Working through past papers is one of the first activities many maths teachers will reach for as a matter of course. The risk of relying on them too much is that students may find themselves falling into a pattern of getting the same questions right and the same questions wrong.

Instead of repeatedly challenging them to take on the five- or six-mark questions of a past paper, class time centred on maths revision may be instead better spent on securing students’ knowledge of the mathematical foundations relevant to the topic at hand.

2. Student-directed revision

Allowing students to focus their GCSE maths revision time on the topics of their choosing, based on what they feel they need to work on most is another common practice.

However, this assumes that GCSE students are themselves the best judges of their own strengths and weaknesses.  

Every GCSE maths student will appreciate receiving positive scores and feedback from their revision activities. A surefire way of making sure that happens will be for them to direct their attention to what they’re already good at. However, this neglects the areas they really need to work on most.

3. Collaborative revision

It might be tempting to set an activity whereby students complete a practice paper in pairs. However, the issue here is that the teacher will often be given little indication as to who did what. Could either student have answered the exam questions correctly working on their own?

4. Student-led lessons

A more extreme instance of practice number 2, this might see each student assigned a particular GCSE maths topic which they then present to the rest of the class.

This is good for encouraging individual students’ engagement with specific mathematical concepts and boosting their confidence. It’s rather less good for the rest of the class.

They’ll likely end up watching a lovingly constructed PowerPoint presentation delivered by a relative novice, while a qualified teacher watches on from a corner of the classroom.

Craig Barton is a maths teacher and the creator of several online maths resources, including diagnosticquestions.com, variationtheory.com and ssddproblems.com. For more details, visit mrbartonmaths.com or follow @mrbartonmaths.

---

Revision checklist and resources

As outlined in the DfE’s Mathematics programmes of study for KS4, your GCSE students’ knowledge will be tested in six distinct subject areas. These are listed below.

Number

Subject content here includes:

• the application of systematic listing strategies
• calculations using roots, and with integer (and fractional) indices
• exact calculations using fractions, surds and multiples of π
• identifying and working with fractions in ratio problems

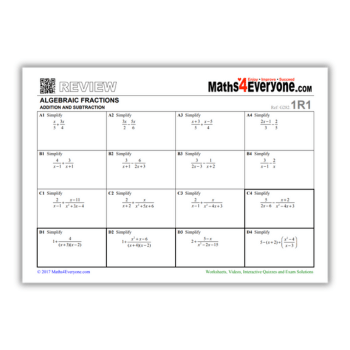
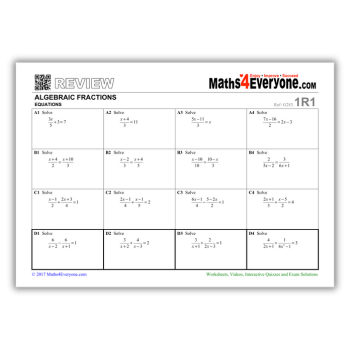
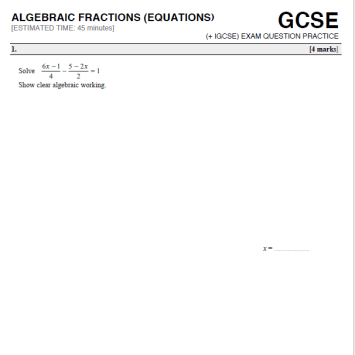
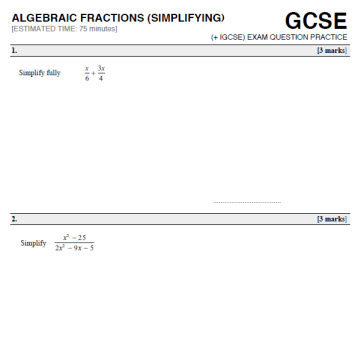
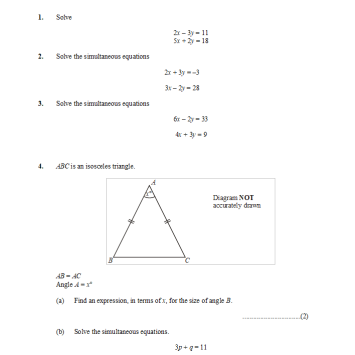
Algebra

Teaching areas covered under algebra include how to:

• simplify and manipulate algebraic expressions
• interpret simple expressions as functions with inputs and outputs
• use the form y mx + c = to identify parallel lines
• identify and interpret roots, intercepts and turning points of quadratic functions graphically
• plot and interpret graphs (including reciprocal graphs)
• recognise and use sequences of triangular, square and cube numbers, along with simple arithmetic progressions, Fibonacci-type sequences, quadratic sequences, and simple geometric progressions.

Ratio, proportion and rates of change

The areas students should be taught include how to:

• compare lengths, areas and volumes using ratio notation and/or scale factors
• convert between related compound units in numerical and algebraic contexts
• interpret the gradient of a straight line graph as a rate of change
• set up, solve and interpret the answers in growth and decay problems

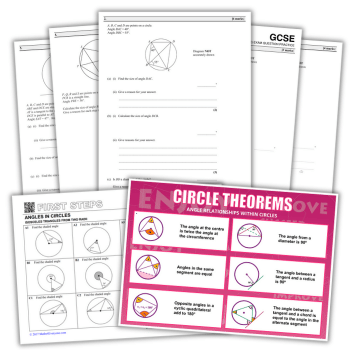
Geometry and measures

Students will be expected to, among other things:

• interpret and use fractional scale factors for enlargements
• identify and apply circle definitions and properties
• construct and interpret plans and elevations of 3D shapes
• interpret and use bearings
• calculate arc lengths, angles and areas of sectors of circles
• calculate surface areas and volumes of spheres, pyramids, cones and composite solids

Probability

Required areas include how to:

• apply the property that the probabilities of an exhaustive set of mutually exclusive events sum to one
• use a probability model to predict the outcomes of future experiments
• calculate the probability of independent and dependent combined events, including using tree diagrams and other representations

Statistics

Students must understand how to:

• infer properties of populations or distributions from a sample, whilst knowing the limitations of sampling
• interpret and construct tables and line graphs for time series data
• apply statistics to describe a population
• use and interpret scatter graphs of bivariate data – while recognising that correlation doesn’t indicate causation, being able to draw estimated lines of best fit, making predictions, interpolating and extrapolating apparent trends, at the same time as being conscious of the risks of doing so

Browse our list of GCSE maths games.