Why we should teach order of operations throughout secondary maths

Regularly revisiting the order of operations as taught in Years 6 and 7 can avoid students slipping up in Year 11, advises Jo Morgan…

- by Jo Morgan
In AQA’s June 2018 GCSE, this relatively straightforward question stumped 61% of higher tier candidates:
Circle the expression that is equivalent to: 3a – a x 4a + 2a.
The options given were:
You can probably guess the most popular answer. Working from left to right incorrectly gives 8a2 + 2a.
This question tested knowledge of the order of operations just as much as it tested algebraic fluency.
Clearly the correct answer was 5a – 4a2 because the multiplication should have been done first.
Both skills in this question are normally taught in Year 7, yet here we have the majority of Year 11 candidates getting it wrong.
Mathematicians will argue that the topic ‘order of operations’ as it’s taught and examined in schools is fundamentally flawed.
‘Real maths’ always uses brackets for clarity, or the context means the order is unambiguous. But nevertheless the order of operations is on the curriculum, and is something many students and teachers struggle with.
Proper priorities
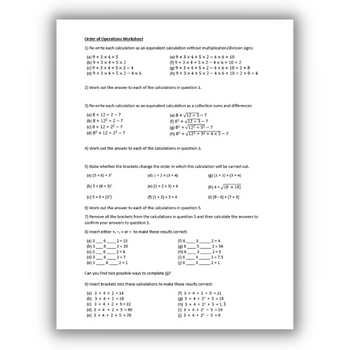
There’s a fairly prevalent gap in teacher subject knowledge here, caused by the misleading mnemonic BIDMAS. This acronym seems to imply that operations should be performed in the order ‘brackets then indices then division then multiplication then addition then subtraction’.
Some graphics used in popular teaching resources reinforce this misconception, resulting in nonsense answers such as 5 – 3 + 2 = 0.
Anyone choosing to use the acronym BIDMAS in their teaching needs to make absolutely clear to their students that division and multiplication hold the same priority and should be dealt with left to right, as should addition and subtraction.
Many teachers have started to move towards alternative mnemonics and graphics in recent years.
Even if well taught in Years 6 and 7, the order of operations must be revisited regularly throughout secondary school. This is vital for embedding the concept in the long term memory and also a good opportunity to develop fluency in other topics.
Say, for example, after an assessment you realise that your Year 10 class would benefit from practice in adding fractions.
Telling students that today’s lesson is on adding fractions may be met with derision. “Oh come on Miss, not fractions. That’s easy, we did it in Year 5”.
In many schools, this is the point where behaviour starts to fall apart. Practice is vital for fluency, but challenge is captivating.
So instead of a set of questions on adding fractions, try something a bit different like this:
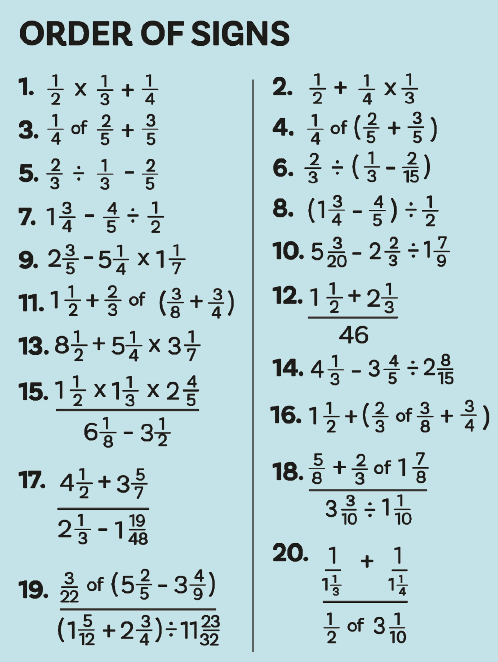
Graded Examples in Arithmetic: Book 1
This is actually an order of operations exercise – something your Year 10 students may well have forgotten if they haven’t been taught it since Year 7 – but it also helps develop fluency in working with fractions. And of course we could do the same thing with decimals.
Algebraic opportunities
Algebra presents yet more opportunities for interleaving. We remind our students of the order of operations when we teach substitution (eg in we square before we multiply). It’s also there when solving equations (eg in we subtract then divide because we’re inversing the operations).
But I wonder how many teachers explicitly teach the order of operations when simplifying expressions. There are very few resources available, which suggests I’m not the only one who has missed this opportunity.
When asked to simplify in their GCSE exam, my students had probably never seen a question like this before. Many would have been bright enough to transfer their knowledge across domains, but nevertheless I’ve decided to cover it more explicitly in future.
Even if a question like this never comes up at GCSE again, it’s still a good opportunity to develop algebraic fluency and further embed knowledge of the order of operations.
You can download a set of questions on using the order of operations when simplifying expressions here.
Jo Morgan is a maths teacher in South London. She writes the website resourceaholic.com and tweets as @mathsjem.