KS4 Chemistry Lesson Plan – BLTs, Accessible Mole Calculations And Chemical Equations

Teaching mole calculations and interpretations of chemical equations to Y10 students can be a challenge – but Dr Joanna Rhodes' tasty ideas will help

- by Dr Joanna L Rhodes
- Vice principal at Leeds Mathematics School

The new GCSE chemistry specifications require mole calculations and interpretation of chemical equations at a similar standard to those we usually begin teaching at AS level. In my experience Key Stage 4 students need a more-visual approach that helps them to see the bigger picture of what the different calculations involving moles are trying to achieve.
Using strategies from elsewhere in the curriculum including computational analogies, food and recipes and writing frames, this lesson aims to give you ideas to crack the challenge of quantitative chemistry in your classroom.
I have just finished teaching the new quantitative chemistry unit at Key Stage 4 to my year 10 students. The concepts and mathematical expectations are more advanced, and have moved closer to A-level chemistry in all respects. However, we are now teaching these concepts to youngsters two years junior in understanding and chemistry experience; the approach I would take with year 12 is very difficult to apply successfully to year 10. This has led me to completely rethink the strategies I use to teach moles, equations, concentration, volume, reacting masses, limiting reagents and yield.
The unit is definitely one of the most challenging we will teach at Key Stage 4 – but with some new ideas and strategies it can still engage and interest all students while preparing the most able with a superb grounding to progress onto A-Level chemistry.
This technical lesson provides strategies, hooks and ideas to introduce students to the main areas of quantitative chemistry, which are addressed in the new program of study. The main aim is to support different learning styles that are more appropriate to year 10 students by drawing on other curriculum areas including food technology, computer science and maths, and on students’ visual and spatial awareness skills.
Starter Activity
Conservation of mass and limiting reactants
If I make a bacon lettuce and tomato sandwich then the mass of the sandwich is equal to the mass of all the ingredients that went into it. The sandwich cannot weigh more than the mass of each ingredient separately, nor can it weigh less than this. If the total mass of the bread, bacon, lettuce and tomato is 250g, then the sandwich will also weigh 250g.
This is the principal of the conservation of mass in chemical equations. Let’s try giving each ingredient a ‘chemical symbol’ so we can see how a chemical equation is put together. If all the ingredients are labelled, this allows us to write the ‘chemical’ equation for making a bacon sandwich as:
2Br + 3Ba + 3L + 2T → Br2Ba3L3T2.
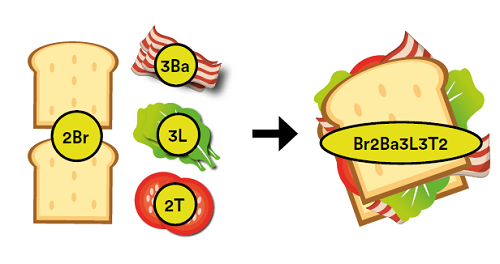
Why not try to create more chemical ‘menus’ of your own that students can use to create their own food equations or ask students to come up with the food equation for their favourite sandwich or meal?
What if I want to make more than one sandwich? Well the equation tells me the ratio of each ingredient that I need. So in the case of the bacon sandwich it is 2 bread: 3 bacon: 3 lettuce: 2 tomato, 2:3:3:2. Ask students to use this ratio to determine the limiting reactant (the one which runs out first) in each of the following cases:
| • | I have 6 slices of bread, 9 rashers of bacon, 6 pieces of lettuce and 6 slices of tomato |
| • | I have 4 slices of bread, 4 slices of bacon, 6 pieces of lettuce and 4 slices of tomato |
| • | I have 2 slices of bread, 6 slices of bacon, 6 pieces of lettuce and 4 slices of tomato. |
Of course I could put different amounts of bacon, lettuce or tomato into my sandwich, but if I did the sandwich would have a different ‘chemical formula’, it would not be the same.
Students should now complete the calculations of Mr (the mass of one mole of a substance) on the GCSE bitesize website to prepare them for the next part of the lesson.
Main Activities
1. The Moles Keyhole
At A-level, I teach reacting masses, concentrations and titrations, gas volumes, mole ratios from chemical equations and theoretical yield as separate concepts and expect students to put each of these together to perform moles based calculations.
This was the first strategy I used to teach year 10; after all the examination specification is also structured in this way with each concept and its associated equations contained within a different specification statement. I discovered however, that students had no idea how to choose which equation to use; they couldn’t put them together to work out reacting masses, volumes and concentrations and they struggled to link the calculations back to the chemical equation or the mole ratio.
It was this challenge that led me to employ the ‘moles keyhole’ for two substances linked by a chemical equation.
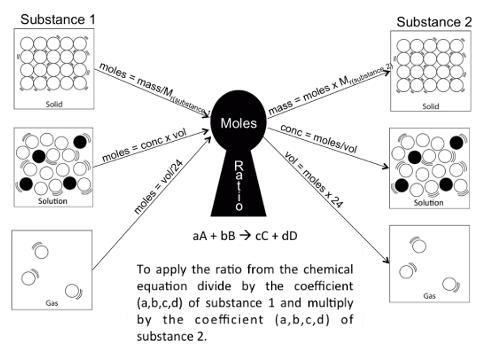
The aim of the moles keyhole is to use a computer programming based approach with inputs and outputs and the mole ratio process in the centre.
To tackle any question, you need to convert a quantitative measurement into an input in moles, you then apply the mole ratio and convert back out of moles to the appropriate quantitative measurement. The system can be summarised as input→process→output.
Examples of how the moles keyhole could be used in calculations include:
i) Calculate the maximum mass of iron that can be made from 500g of iron oxide according to the equation: 2Fe2O3 + 3C → 4Fe + 3CO2
| Step 1 (input) | Convert 500g of iron oxide into moles using the input equation moles = mass/Mr(Fe2O3) |
| Step 2 (process) | Apply the mole ratio of 2:4 to work out the moles of iron formed |
| Step 3 (output) | Convert the moles of iron into mass using the output equation mass = moles x Mr(Fe) |
ii) Calculate the maximum volume of hydrogen gas that is produced when 15g of magnesium ribbon reacts with an excess of hydrochloric acid according to the equation: Mg + 2HCl → MgCl2 + H2
| Step 1 (input) | Convert 15g of magnesium into moles using the input equation moles = mass/Mr(Mg) |
| Step 2 (process) | Apply the mole ratio of 1:1 to work out the moles of hydrogen gas formed |
| Step 3 (output) | Convert the moles of hydrogen into volume using the output equation vol = moles x 24 |
iii) 25cm3 of H2SO4 is completely neutralised by 14.5cm3 of 0.5mol/dm3 NaOH. Calculate the concentration of H2SO4. The equation for the reaction is H2SO4 + 2NaOH→ Na2SO4 + 2H2O.
| Step 1 (input) | Convert 14.5cm3 (14.5 x 10-3 dm3) of 0.5mol/dm3 NaOH into moles using the equation moles = conc x volume |
| Step 2 (process) | Apply the mole ratio of 2:1 to work out the moles of H2SO4 that have reacted |
| Step 3 (output) | Convert the moles of sulphuric acid into concentration using the output equation conc = moles/vol(H2SO4) |
iv) 650g of Ti is formed from the reaction of 2000g of TiCl4 according to the equation TiCl4 + 2Mg → Ti + 2MgCl2 . What is the percentage yield of the reaction?
| Step 1 (input) | Convert 200g of TiCl4 into moles using the equation moles = mass/Mr(TiCl4) |
| Step 2 (process) | Apply the mole ratio of 1:1 to work out the theoretical moles of Ti that could be formed |
| Step 3 (output) | Convert the moles of Ti into mass using the output equation mass = moles x Mr (Ti) |
| Step 4 (yield) | Calculate the percentage of the actual mass formed (650g) compared to the theoretical maximum that you have just calculated in step 3 |
2. Balanced equations from moles of substance
The moles keyhole approach requires the mole ratio from the chemical equation to be known, or a balanced chemical equation to be given. Students may be required to produce the balanced chemical equation using reacting masses. Using a writing frame for this type of calculation may help to make the problem more visual for learners.

Examples of how the calculation writing-frame can be used to produce balanced equations:
i) 8.1g of zinc oxide (ZnO) reacts completely with 0.60g of carbon to form 2.2g of carbon dioxide and 6.5g of zinc. Use these masses to construct the balanced symbol equation.
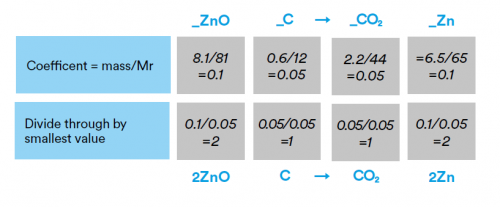
So the equation is 2ZnO + C→ CO2 + 2Zn
ii) In a chemical reaction, 36g of magnesium (Mg) reacts with 98g of phosphoric acid (H3PO4) to make 131g of magnesium phosphate (Mg3(PO4)2) and 3g of hydrogen (H2). Use these masses to construct the balanced symbol equation.
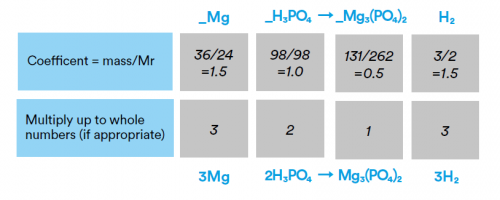
So the equation is 3Mg + 2H3PO4 → Mg3(PO4)2 + 3H2
Summary
The website gcsescience.com contains examples of moles calculations between mass, volume, molecules and concentration as well as conversions and example calculations. Students should log on at home or in school to complete the revision questions as directed by the teacher.
Extension activity
Gas volumes
The equations for volume given in the moles keyhole example are based on a temperature of 25°C and atmospheric pressure.
As an extension for your more able, or gifted and talented students, introduce the ideal gas equation: pV=nRT where p is the pressure in Pa, V is the volume in m3, n is the number of moles, R is the gas constant 8.314 J K-1mol-1 and T is the temperature in K.
Also give students a number of exercises to practice converting between cm3 and dm3 and m3 and between temperature in °C and K.
Then, set them the following problem:
“Two students have been given the task of filling a balloon with a volume of 15dm3 of carbon dioxide. One of them decides to connect the balloon to a flask containing dry ice and the balloon inflates as the dry ice evaporates until 15dm3 is collected and the balloon is sealed. The other student connects the balloon to a flask containing marble chips (calcium carbonate) and hydrochloric acid, again 15dm3 is collected and the balloon is sealed”. Later the students measure the mass of their balloons and are surprised to discover it is different. Explain why using calculations to support your answer.
The solution to the problem is achieved by calculating n for constant pressure and volume but with a temperature of -78.5°C (194.5K) for the carbon dioxide collected from cold dry ice and a temperature of 25°C (298K) for the carbon dioxide collected from the reaction at room temperature (which may even be a little bit higher as the reaction is exothermic).
The masses calculated using these values and atmospheric pressure (101325 Pa) will be approximately 41g for the cold balloon and 27g for the warm balloon.
For further opportunities to practice their moles calculations students could be directed to complete this online quiz from the Royal Society of Chemistry.
Download this lesson plan as a PDF here.









